Day: November 2, 2019

Can an irrational number raised to an irrational power be rational?

~ squarishbracket ~ Leave a comment

There’s a wonderful proof that yes, indeed, this is possible, and it goes as follows:

Let’s consider the number \sqrt 2 ^ {\sqrt 2} . This number is either rational or irrational. Let’s examine each case.

Case 1: \sqrt 2 ^ {\sqrt 2}  is rational.

Recall that \sqrt 2  is irrational. So if \sqrt 2 ^ {\sqrt 2}  is rational, then we have proven that it’s possible to raise an irrational number to an irrational power and get a rational value. Done!

Case 2: \sqrt 2 ^ {\sqrt 2}  is irrational.

In this case, \sqrt 2 ^ {\sqrt 2}  and \sqrt 2  are both irrational numbers. So what if we raise the \sqrt 2 ^ {\sqrt 2}  to the power of \sqrt 2 ?

\left( \sqrt 2 ^ {\sqrt 2} \right) ^{\sqrt 2} =  \sqrt 2 ^ {\sqrt 2 \cdot \sqrt 2} = \sqrt 2 ^ 2 = 2

So in this case, we have again found a pair of irrational numbers such that one raised to the power of the other is a rational number! Proof complete!

✯✯✯

One thing that’s fun about this proof is that the result is pretty surprising. I would not have guessed a priori that you could get a rational by raising one irrational to another; it just seems like irrationality is the type of thing that would be closed under ordinary arithmetic operations.

But an even cooler thing is that it’s a non-constructive proof. By the end of the proof, we know for sure that there is a pair of irrational numbers such that one raised to the other gives us a rational number, but we have no idea whether it’s (\sqrt 2 , \sqrt 2 ) or (\sqrt 2 ^ {\sqrt 2} ,\sqrt 2 ).

(It turns out that it’s the second. The Gelfond–Schneider theorem tells us that for any two non-zero algebraic numbers a and b with a ≠ 1 and b irrational, the number ab is irrational. So \sqrt 2 ^ {\sqrt 2}  is in fact irrational.)

Now, most mathematicians are totally fine with non-constructive proofs, as long as they follow all the usual rules of proofs. But there is a branch of mathematics known as constructive mathematics that only accepts constructive proofs of existence. Within constructive mathematics, this proof is not valid! 

Now, it so happens that you can prove the irrationality of \sqrt 2 ^ {\sqrt 2}  by purely constructive means, but that’s besides the point. To my eyes, the refusal to accept such an elegant and simple proof because it asserts a number’s existence without telling us exactly what it is just looks a little silly!

Along similar lines, here’s one more fun problem.

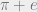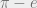Are \pi + e and \pi - e transcendental?

We know that \pi and e are both transcendental numbers (i.e. they cannot be expressed as the roots of any polynomial with rational coefficients). But are \pi + e and \pi - e both transcendental?

It turns out that this amazingly simple sounding problem is unsolved to this day! But one thing that we do know is that it can’t be that neither of them are transcendental. Because if this was the case, then their sum (\pi + e) + (\pi - e) = 2 \pi would also not be transcendental, which we know is false! So we know that at least one of them has to be true, using a proof that doesn’t guarantee the truth of either of them! Cool, right?